
Math-Made Materials
Carnegie Mellon Mathematicians Unravel the Complex Laws Underlying Materials Design
by Amy Pavlak
Civilizations have been manipulating materials since Bronze Age metallurgists stirred together molten copper and tin to make tools and weapons that were harder and more durable than those made of copper alone. Even though they didn’t know the chemistry or the physical principles that made bronze stronger than copper, our ancestors used trial and error to create materials that suited their needs. We’ve come a long way in the last 5,000 years. By uncovering many of the laws of nature that govern physical phenomena, we’ve increased our ability to engineer materials with more precision than ever before.
But in spite of these advances, materials design can still be hit or miss—materials scientists might mix metals together to create an alloy, measure its properties, find it lacking, go back to the lab to tinker with the proportions, measure the modified alloy’s properties, and do it all again until they discover an alloy with properties that make it interesting and commercially relevant. To avoid such trial-and-error, new approaches are needed to reveal the underlying physical principles that govern how the two, three or even four materials meld together to form an alloy.
“Materials are complicated enough that we still don’t have all the predictive laws that we need to truly engineer,” said Katayun Barmak, Philips Electronics Professor at Columbia University and adjunct professor of materials science and engineering at Carnegie Mellon. “We cannot yet sit at a computer, design a material and be able to build it to do exactly what we designed it to do. We’re not there yet.”Mathematicians can help engineers get there. They can use math to search for—and rigorously explain—the theories and laws that underlie complex physical phenomena. For more than 20 years, mathematicians in CMU’s Center for Nonlinear Analysis (CNA) have been creating and using mathematical tools to understand materials—from batteries and fuel cells to liquid crystals and shape memory alloys.
“The exceptional group of applied mathematicians in the CAN are pushing the frontier of mathematics relating to materials science,” said Tom Bohman, the Alexander M. Knaster Professor and Head of the Department of Mathematical Sciences. “They are pioneers in eroding the barriers between fields and in so doing have made many contributions to the understanding of materials.”
Carnegie Mellon’s preeminence in the field of applied mathematics was recently acknowledged with a five-year, $5 million grant from the National Science Foundation’s Partnerships for International Research and Education (PIRE) project, a program that supports international activities across all NSF-supported disciplines. Carnegie Mellon’s PIRE is led by the Mellon College of Science Professor of Mathematics Irene Fonseca, who is currently president-elect of the Society for Industrial and Applied Mathematics, an international professional society for applied mathematicians with more than 13,000 individual members and almost 500 institutional members. The PIRE project includes faculty, postdocs and students from 10 universities in the United States and Europe who are working together to address issues in applied mathematics and mechanics that arise from the materials sciences.
“These very applied and very contemporary problems are becoming more and more complex, so you need to bring different types of expertise to the table,” Fonseca said. “What we do on our end is we talk with our colleagues in neighboring disciplines to find out what the challenges are. Then we work to develop new mathematics to address a particular problem they may be facing.”
Mathematics at the Nanoscale

Irene Fonseca (left) and Giovanni Leoni develop new mathematical tools to address issues that arise from the materials sciences.
Fonseca and her close collaborator Mathematical Sciences Professor Giovanni Leoni lately have been interested in understanding the mathematics behind the formation of quantum dots, nanometersized semiconductor crystals that glow when exposed to an electric current or light. Quantum dots have tremendous potential for use in a new generation of lasers, LEDs, telecommunication devices and information storage technologies. But before quantum dots can be used for these commercial applications, scientists must gain better control over the fabrication process—and that requires understanding the fundamental laws that govern the dots’ formation.
One way that scientists grow quantum dots is by depositing a very thin layer of semiconductor, called a film, onto a substrate that is made of a different type of semiconductor material. At the atomic level, the two materials have different lattice structures that don’t match up. At first the film’s atoms try to rearrange to fit with the substrate’s atoms. But as more and more film is added, the film’s atoms can no longer conform to the substrate. As the atoms clash at the interface, stresses and strains arise. Once the film reaches a critical thickness, it needs to relax and release some of the stress. It does this by separating into islands that pop up into the third dimension.
The islands, which are the quantum dots, start out looking like a half-pyramid, but as more film is added, the islands start taking on different shapes—pyramids, domes and finally barns. Each shape has its own distinct electronic and optical properties.
“Materials scientists want to control the process of quantum dot formation to create the exact shapes that they want,” said Leoni. “To do this they need to be able to predict how quantum dots form and how the shapes evolve as more film is added.”
To describe the process, materials scientists develop physical models based on observations from experiments and numerical simulations. Their objective is to recreate in a computer model what they see in their experiments. A computer model that takes into account all of the laws of physics would require massive amounts of computing power to run, so scientists throw away a lot of the mathematical and physical details to build manageable models. Fonseca and Leoni are using rigorous mathematical tools to prove that the simplified models are still valid.
In the case of quantum dots, Fonseca and Leoni’s goal was to fill in the model by identifying the underlying laws and theories that explain and predict what drives the islands’ shape changes. Together with collaborators and postdocs, they focused on the interaction and balance of bulk and surface energies at the interface between the two materials.
The rigorous analytical treatment of this problem is particularly challenging, according to Fonseca and Leoni, because numerical and experimental results show the formation of cusp singularities on the profile of the film, which disrupt its smoothness. Explaining the cusps’ presence mathematically requires introducing novel methods and techniques, which lie outside the “classical” framework of regular fields, that allow the presence of such singularities and discontinuities.
Their results explain and validate experimental observations of a finite number of cusps in a germanium film on a silicon substrate, and prove that the shape of the island evolves with the size.
“Now we can give materials scientists a mathematical theorem that tells them exactly how much film causes the shape to evolve from a pyramid to a dome, from a dome to a barn. And we know exactly what the transitions are,” Fonseca said.
Revealing the Natural Order of Things

David Kinderlehrer, Alumni Professor of Mathematical Sciences and professor of materials science and engineering, shows off the honeycomb-like crystals that form a piece of agate. This rock, like most natural and made-made materials, is composed of aggregates of crystals called grains.
While Fonseca and Leoni are interested in what happens at the interface between different materials as they change and evolve, Alumni Professor of Mathematical Sciences David Kinderlehrer and Mathematical Sciences Professor Shlomo Ta’asan focus on the interfaces that are present within a material. For the past 15 years they have worked closely with materials scientists on a quest to understand a phenomenon that occurs in nearly every engineered material.
Most materials—from the wires in a computer to the beams of steel in buildings and bridges—are composed of aggregates of crystals called grains. Like a jigsaw puzzle whose pieces don’t quite fit, the millions of grains that form a piece of metal, for example, don’t form a perfect, seamless structure. The boundaries between the grains can be mismatched, exhibiting varying angles, orientations and energies. The types of boundaries between the grains affect a wide range of a material’s properties and, ultimately, its performance and durability.
In the late 1990s, a team of CMU materials scientists including Greg Rohrer, Tony Rollett and the late William Mullins were studying grain boundaries. They were—and many materials scientists still are—interested in learning more about grain boundaries so that they could manipulate them to produce a material with a desired set of properties. But they first had to understand why and how the grains and the boundaries evolve under various conditions used in materials manufacturing, like heat or pressure. The team of CMU materials scientists approached Kinderlehrer and Ta’asan and asked them to collaborate on the project. They’ve been working together ever since.
“It’s very rare for materials scientists and mathematicians to collaborate,” said Greg Rohrer, the W.W. Mullins Professor and Head of the Department of Materials Science and Engineering at CMU. “There are some differences in concepts and methods that are hard to bridge. David and Shlomo have always made that easy for us.”
The interdisciplinary team, which also included materials scientist Katayun Barmak and postdocs Eva Eggeling, Maria Emelianenko, Yekaterina Epshteyn and Richard Sharp, developed a large-scale computer simulation of the evolution of grain boundaries. This required an innovative use of partial differential equations for describing the misorientation, rotation and energy at the millions of interfaces in a chunk of material.
“These materials are very dirty. There are defects, perturbations in the lattice structure that shouldn’t be there. All kinds of things are going on,” Kinderlehrer said. “There wasn’t a theory which prescribed how the network is going to behave as it evolves. That’s what we had to find.”
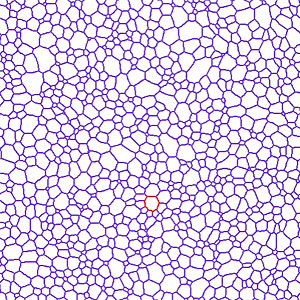
Frame from a large-scale computer simulation of the evolution of grain boundaries. As the model system evolves under heat or pressure, for example, some of the grain boundaries get bigger, others get smaller, while some of the boundaries—and thus the grains—disappear completely.
By harvesting vast amounts of data from the simulation, the team observed that some of the grain boundaries get bigger, others get smaller, while some of the boundaries — and thus the grains — disappear completely. The system eliminates the highenergy boundaries to arrive at a more orderly, low-energy state. The team developed a completely new theory, which uses the mass transport method for a well-known diffusion equation, to describe how the grain boundary network evolves into this ideal distribution over time.
“Using the mass transport method for the Fokker-Planck Equation was a flash of inspiration. Most flashes are misfires,” Kinderlehrer quipped.
In this case, his mathematical insight was dead-on.
“Experiments over the past decade have shown that there are more low-energy boundaries than high-energy boundaries, but quantifying this and understanding how it happens is an enormous challenge,” Barmak said. “Our results provide another piece of the puzzle and are a huge step forward.”
This information is valuable to materials scientists who study the polycrystalline microstructure of materials. When they want to engineer a metal or a ceramic to have certain properties, they are generally looking to manipulate the boundaries. For example, they may want to design a semiconductor with a smooth distribution of boundaries to make it conduct electricity more efficiently.
“Mathematics is the language of materials science, and science in general,” said Richard D. James, the Distinguished McKnight University Professor in the Aerospace Engineering and Mechanics Department at the University of Minnesota and a member of the PIRE project. “To develop a concept, it needs to be put into equations, and you can make predictions from those equations on things that you wouldn’t know otherwise. There would be no amount of experiments you could do that would give you that information.”
Crossing New Boundaries
Exploring what happens at the boundaries within materials or at the interface between two materials is Kinderlehrer, Ta’asan, Fonseca and Leoni’s specialty. But the theories they develop aren’t just useful for materials science applications.
“When you study a problem, you don’t just study that specific problem. You try to put it in a framework which is a little more general or as general as you can be,” Leoni said. “That’s why some of the mathematical models we develop can be used for different problems.”
Fonseca and Leoni are currently adapting the mathematical models they and their CNA colleagues developed to explain materials’ behavior to address problems in image segmentation, image recognition and image reconstruction, such as restoring color to a faded photograph or even to a fresco.
“Often the tools and the theories that you develop for a particular application turn out to be useful in completely different areas,” Fonseca added. “That is the beauty of mathematics.”